
Important Properties of Two-Locus Model

Two Neutral Loci
- Disequilibrium decays at a rate r every generation.
- Allele frequencies do not change.
- Chromosome frequencies do change as the disequilibrium decays.
One Neutral Locus; One Selected Locus
- Selected locus evolves as in the one-locus selection model.
- Neutral locus also changes in frequency at a rate that depends on the disequilibrium between the loci (=HITCHHIKING).
- If B2 is favorable and D is positive, A2 increases in frequency.
- If B2 is favorable and D is negative, A2 decreases in frequency.
- The extent of hitchhiking will be large only when r is small (D decays slowly).
Two Selected Loci
- Not fully analysed.
- It is postulated that with pure directional selection the favorable genotype rises to fixation (NOT PROVEN).
- With overdominance at both loci,
- If fitnesses at the two loci add together to determine fitness (ADDITIVE MODEL), the one-locus equilibrium predicts the two locus equilibrium.
- If fitnesses at the two loci multiply together to determine fitness (MULTIPLICATIVE MODEL), the one-locus equilibrium predicts the two locus equilibrium ONLY when r is large. When r is small, the population approaches a polymorphic equilibrium where linkage disequilibrium is maintained.
- (Similar results are observed with a SYMMETRIC MODEL of fitness.)
- Cycling can occur under some fitness schemes.
- Linkage disequilibrium does not always decay to zero.
- Mean fitness at equilibrium is highest at r=0 (or the same for all r).
- Mean fitness can decline over time!!

Generating Novel Variation: Mutation

Mutation:
"Spontaneous change from allele A to allele a" (Hartl & Clark) due to
- basepair substitutions
- deletions
- insertions
- inversions
- translocations
Rates of Mutation
- Coat color mutants in mice (Russell 1963; Schlager and Dickie 1971)
- 11.2 10-6 per gene per generation (wildtype to mutant)
- 2.5 10-6 per gene per generation (mutant to wildtype)
- Allozyme mutants in Drosophila (Voelker 1980)
- 1.3 10-6 per gene per generation (wildtype to allozyme with different mobility)
- 3.9 10-6 per gene per generation (wildtype to inactive allozyme)
- Polypeptides in humans (Neel et al 1986)
- 5.6 10-6 per gene per generation
 The genome of an organism is not faithfully replicated from generation to generation, but changes in eukaryotes at an approximate rate of 10-6 - 10-5 per gene per generation or 10-9 - 10-8 per basepair per generation.
The genome of an organism is not faithfully replicated from generation to generation, but changes in eukaryotes at an approximate rate of 10-6 - 10-5 per gene per generation or 10-9 - 10-8 per basepair per generation.
 Given the large number of basepairs in the genome (3.3 109 in the human genome), a fairly large number of new mutations is expected somewhere within the genome each generation.
Given the large number of basepairs in the genome (3.3 109 in the human genome), a fairly large number of new mutations is expected somewhere within the genome each generation.

One-Locus Mutation Model (No Selection)
 Let mutations happen at meiosis:
Let mutations happen at meiosis:
-
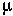 is the mutation rate from A1 to A2
is the mutation rate from A1 to A2
-
 is the mutation rate from A2 to A1
is the mutation rate from A2 to A1
Let p[t] be the frequency of A1 in the gamete pool after meoisis.
Since there is no selection, p[t] will also be the frequency of A1 in the adults that are about to undergo meiosis.
In the next generation,
 The only equilibrium of this equation is when p[t+1]=p[t]=p*:
The only equilibrium of this equation is when p[t+1]=p[t]=p*:

p*=0 and p*=1 are NOT equilibria. Fixation is not stable when mutations recur.

One-Locus Mutation Model (No Selection)
 How rapidly does the population approach this equilibrium?
How rapidly does the population approach this equilibrium?

The population approaches equilibrium, but at a rate of only 1
-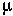 -
- .
.
 VERY SLOW!
VERY SLOW!

One-Locus Mutation Model (No Selection)

Example
With 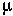 =
=
 = 10-6,
at equilibrium p*=1/2.
= 10-6,
at equilibrium p*=1/2.
With a starting frequency of p[0]=0
- p[10,000]=0.0099
- p[100,000]=0.0906
- p[1,000,000]=0.4323
Approximately, 1/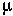 generations must pass before the population nears equilibrium!
generations must pass before the population nears equilibrium!
This occurs over such a long time frame that other forces such as
viability selection (even very weak selection) or simply sampling error
in finite populations are likely to overwhelm evolution of the system to
 .
.

One-Locus Mutation Model With Selection
 Mutations often cause changes in fitness.
In the vast majority of cases, these changes are deleterious (Examples: human disease-causing mutations).
Only rarely will a mutation produce a more fit individual.
We will focus on the dynamics of deleterious mutations.
Mutations often cause changes in fitness.
In the vast majority of cases, these changes are deleterious (Examples: human disease-causing mutations).
Only rarely will a mutation produce a more fit individual.
We will focus on the dynamics of deleterious mutations.
Model of Mutation-Selection Balance
Let selection act upon diploid individuals with relatives fitnesses equal to:

Life Cycle:

The allele frequencies in the adults (before mutation) are given by the recursion equations in the one-locus model without selection from last week:


One-Locus Mutation Model With Selection
 Now meiosis and mutation occur to produce the next generation of gametes:
Now meiosis and mutation occur to produce the next generation of gametes:

The recursions with mutation and selection are therefore:

Assuming selection is stronger than the mutation rate, the population will move
rapidly towards fixation on A1 as if there were no mutation.
The fixation states are not equilibria, however:
- If q=0, q'=
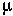
- If p=0, p'=

Instead the population will tend to an equilibrium that solves:

Using Mathematica, we can show that this does not factor.
Instead it yields an ugly cubic equation.


One-Locus Mutation Model With Selection

Definition
If all fixation states are unstable then there must be a PROTECTED POLYMORPHISM. (Warning: This is only strictly true in a one dimensional system, since cycling could occur if there were multiple dimensions.)
Approximate Solution
Assume that the mutation rates are much smaller than the selection coefficients (both hs and s).
The population will move rapidly towards fixation on A1 as if there were no mutation,
but will not quite reach fixation since mutation "protects" the system from fixation.
We therefore assume that q is small (of the order of the mutation rate).
We then perform a Taylor series on the cubic equation, keeping only the leading order terms.

We find that

Compare to exact solution from Mathematica.
(Special case: If there is complete recessivity (h=0) so that only the A2A2 genotype is selected against, then the above equilibrium no longer exists and the population tends instead to:



One-Locus Mutation Model With Selection

Stability
If we perturb the population a little (p[t]=p*+ ), does it return to p*?
), does it return to p*?
Again, write the recursion equation p[t+1] as f(p[t]) and do a
Taylor approximation:

Note that f(p*) is p*.
Therefore, p[t+1] will be closer to p* than p[t] only
if the derivative of f(p*) is less than one.
 When the derivative is less than one, the equilibrium is locally stable.
When the derivative is less than one, the equilibrium is locally stable.
In this case, the derivative is approximately 1-hs and so the equilibrium with
p*=1-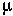 /hs is stable.
/hs is stable.


One-Locus Mutation Model With Selection

Mean Fitness
The mean fitness in the one-locus model with mutation and selection equals:

This is maximized at q=0 (where the mean relative fitness equals 1).
At the mutation-selection balance, the mean fitness equals:

Point 1 The mean fitness at equilibrium is not the maximum possible.
Point 2 Mean fitness can decrease over time. Whenever the population starts nearer q=0 than
q*=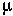 /hs, the mean fitness will decline towards 1-2
/hs, the mean fitness will decline towards 1-2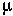 .
.
Point 3 The reduction in mean fitness due to mutation is not that great
at one locus alone, but it may be substantial with mutations occurring throughout the
genome (= "Mutation Load").
Mutation Load
If there are no fitness interactions among genes (no epistasis), then the average fitness of an individual would be (1-2 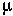 )# loci
)# loci  e-2
e-2 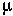 (# loci) = e-U, where U is the sum total deleterious mutation rate in a diploid genome.
(# loci) = e-U, where U is the sum total deleterious mutation rate in a diploid genome.
What is U?
A classic experiment by Mukai et al. (1972) estimated the genome-wide deleterious mutation rate by measuring the decline over time in viability in Drosophila melanogaster.
Mukai relaxed selection by choosing at random only one individual male and three females to mate each generation (little variation in fitness, so little natural selection).
He then measured the average viability of individuals made homozygous for chromosomes that had accumulated mutations in his experiment:

(From Mukai et al. 1972)
The mean viability declined over time at a rate of U s (the mutation rate times the average effect of each mutation).
Mukai also used a formula for how the variance should change over time to disentangle U and s:
 U > 0.6 per diploid genome per generation.
U > 0.6 per diploid genome per generation.
Current estimates of U for multicellular animals and plants are roughly 0.2-2.0 (Lynch and Walsh 1998), but more data are sorely needed.
These estimates suggest a major fitness cost:
U | Average fitness = e-U | Mutation load (= 1-fitness) |
0.2 | 0.82 | 0.18 |
0.6 | 0.55 | 0.45 |
2.0 | 0.14 | 0.86 |
Therefore, using Mukai's estimate for Drosophila, fitness would be reduced by 45% due to the continual onslaught of new mutations!! [If assumptions are accurate.]

Review of Properties of One-Locus Model

Selection; No mutation
- Mean relative fitness is maximized.
- Population tends toward fixation unless there is overdominance.
No Selection; Mutation
- Fixation states are unstable and population tends towards an internal polymorphism.
- The rate of approach to this polymorphism is, however, extremely slow.
Selection; Mutation
- Fixation states are no longer equilibria.
- With directional selection favoring A1, the population tends to a mutation-selection balance fairly near fixation on A1.
- Mutation reduces the average fitness below its maximum (but introduces the variance critical for further evolution).
 Back to Zoology 500 home page.
Back to Zoology 500 home page.






 The genome of an organism is not faithfully replicated from generation to generation, but changes in eukaryotes at an approximate rate of 10-6 - 10-5 per gene per generation or 10-9 - 10-8 per basepair per generation.
The genome of an organism is not faithfully replicated from generation to generation, but changes in eukaryotes at an approximate rate of 10-6 - 10-5 per gene per generation or 10-9 - 10-8 per basepair per generation.
 Given the large number of basepairs in the genome (3.3 109 in the human genome), a fairly large number of new mutations is expected somewhere within the genome each generation.
Given the large number of basepairs in the genome (3.3 109 in the human genome), a fairly large number of new mutations is expected somewhere within the genome each generation.


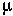 is the mutation rate from A1 to A2
is the mutation rate from A1 to A2
 is the mutation rate from A2 to A1
is the mutation rate from A2 to A1





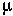 -
- .
.
 VERY SLOW!
VERY SLOW!


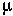 =
=
 = 10-6,
at equilibrium p*=1/2.
= 10-6,
at equilibrium p*=1/2.
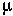 generations must pass before the population nears equilibrium!
generations must pass before the population nears equilibrium!
 .
.









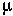











 ), does it return to p*?
), does it return to p*?

 When the derivative is less than one, the equilibrium is locally stable.
When the derivative is less than one, the equilibrium is locally stable.
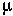 /hs is stable.
/hs is stable.





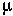 /hs, the mean fitness will decline towards 1-2
/hs, the mean fitness will decline towards 1-2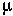 .
.
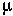 )# loci
)# loci  e-2
e-2 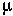 (# loci) = e-U, where U is the sum total deleterious mutation rate in a diploid genome.
(# loci) = e-U, where U is the sum total deleterious mutation rate in a diploid genome.

 U > 0.6 per diploid genome per generation.
U > 0.6 per diploid genome per generation.

